Basic rules
- The result of reading the statement is usually pure math model. If story helps to build correct understanding, you can keep it, but still try to discard as many unnecessary details as possible.
- Imagine you want to tell the problem to someone else. What parts you want to tell? (According to my PM, this rule won't help you).
- Shorter = better.
- Simpler = better.
- Limitations are part of problem statement. Especially small limitations, because for small data you can try all the possibilities.
- Samples are part of problem statement. After building math model, check that you model working correct on samples, at least on small samples where you can check everything fast.
- Notes are part of problem statement.
- Try to find familiar patterns, maybe objects you already know something about. If you are given some connected graph with exactly one simple path between each pair of vertices, it's called tree. 4 letters instead of 12 words, see?
- Try even harder to spot something strange, something you not expecting. That probably will be the cornerstone of the problem.
- If there is some part of the statement you don't like, try to change that to something you like. Start with understanding the object, then simplify it. There are some problems which can be completely solved by this technique.
- If the model you get is very big, try to split it into some pieces. It would be great if pieces are independent, like 'solve problem1, then use its answer as input to problem2 and so on'.
- On first stages it can be useful to write your new statement. On paper. By hand.
- Problemsetters do not write random things in statements. But why would you believe me, since I'm a bad problemsetter? I don't know, maybe you shouldn't.
Some examples
Assumed workflow: read the statement, try to understand and simplify it using the rules above. You don't have to read "solution" parts, but I warn you that my concept of reading statements works. I mean, "statement" parts will often contain huge hints to solution.
In most cases I won't write step-by-step explanations how I get the final version. You can say that that's not how teaching works. I can say that you don't want to study. I'll win, because that's my blog.
Given undirected graph, find its spanning tree with minimal diameter.
Diameter has exactly one center. Let's fix the center, it's either vertex or middle of edge. For fixed center the tree with minimal diameter is a tree with minimal height if it's rooted at center. Run BFS to find it.
Different math models will lead you to different solutions
Well, only if you have some patterns of how you should solve problems. And you should have them, patterns are good, they're the fastest way to solve problems.
Eliminating things you don't like can solve the problem
Given a tree, all vertices are initially white. Two players, Red and Blue, take turns coloring white vertices (surprise) red and blue. The game ends when there are no white vertices left. The score of the game is CR - CB, where CR is a number of connected components on red vertices, and CB is a number of connected components on blue vertices. Red wants to maximize the score, Blue wants to minimize it. Find the score if both play optimally. n ≤ 2·105.
The statement is clear, but we don't like the formula. Especially we don't like Ci, because they change unpredictably. What can we say about connected components after deleting some vertices of a tree? Well, they are all trees themself. What we know about trees? Number of vertices is exactly 1 bigger than number of edges. OK, so number of components in a forest is exactly number of vertices minus number of edges. We can now rewrite the formula. CR - CB = (VR - ER) - (VB - EB) = EB - ER + (VR - VB). Value in brackets is constant, it is 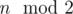 . So now we play not for components, but for edges. Better, but still not obvious.
. So now we play not for components, but for edges. Better, but still not obvious.
We control vertices, can we somehow express Ei using only vertices? Do we have any equations expressing number of edges through vertices? Yes, we have. 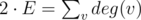 . But for calculating ER we should sum only degR(v), the number of red neighbors. That's bad, but let's try anyways.
. But for calculating ER we should sum only degR(v), the number of red neighbors. That's bad, but let's try anyways.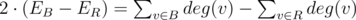 . Turns out this is true, because all RB-edges cancel out.
. Turns out this is true, because all RB-edges cancel out.
So, formula for score is 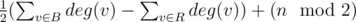
Now it is easy to see that each player should take vertex with smallest degree available at the moment.
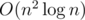 is standard and
is standard and  is possible, probably only with library code :)
is possible, probably only with library code :)

No comments:
Post a Comment